INTRODUCTION
The peninsula of Lezíria de Vila Franca de Xira is an agricultural area of alluvial origin, approximately 10 km northeast of Lisbon (Portugal). It is surrounded by River Tejo, its estuary, and by River Sorraia. The peninsula lies 1 to 2 m above sea level, with saline groundwater arising from the estuarine tides, which salinity is close to that of the sea, with an electrical conductivity (EC) of 40-50 dSm-1 (Rodrigues et al., 2019). Climate projections for the Mediterranean region show a decrease in precipitation, increase in mean temperature, and increase in sea water level. These conditions potentiate the accumulation of salts in the soil profile, which may compromise its agricultural use.
Hydrus-1D is a modelling tool which has been found to accurately model the soil water and salts dynamics (Kanzari et al., 2018; Ramos et al., 2011). In this study, Hydrus-1D was used to evaluate the influence of climate change projections on soil salinity and water content, in a rainfed spontaneous pasture in the south of Lezíria.
MATERIAL AND METHODS
Study site
The site is a rainfed spontaneous pasture dominated by Poaceae. The soil is classified as a Solonchak, according to the Harmonized World Soil Database (Fischer et al., 2012). The soil texture is relatively uniform along the soil profile and is classified as silty-clay. The site is located 2 m above the sea and at about 2.5 km from the estuary of the River Tejo. Primary salinization occurs due to the influence of the marine water from the estuary in groundwater.
Calibration of the Hydrus-1D model
Hydrus-1D is a public domain modelling tool for analysis of one dimensional water flow and solute transport in variably saturated porous media (Simunek et al., 2018).
Five soil layers to a depth of 1.5 m were considered (average depths of 0.15, 0.45, 0.75, 1.05, and 1.35 m). For the model calibration, soil samples were collected from the five soil layers, between May 2017 and October 2018, in a total of 20 samples. The soil volumetric water content (θ) was determined from the soil gravimetric water content (θ w ) and the dry bulk density. The electrical conductivity of the soil saturated paste (EC e ) was determined with a conductivity meter in the extract collected with suction filters from the saturated soil paste. The electrical conductivity of the soil solution, EC sw , was calculated according to the following equation,
where SP, is the gravimetric water content of the saturated soil paste (Rhoades et al., 1989). In Hydrus-1D the van Genuchten-Mualem model was selected to calculate the water transport, using the saturated and residual water content (θ s and θ r ), the saturated hydraulic conductivity (K s ), the inverse of the air-entry value (α), the pore-size distribution index (n), and the pore connectivity (l). θ s and θ r were obtained from the particle size distribution using pedotransfer functions developed by Ramos et al. (2014), which were found to be well-suited for the soils in this region. The equilibrium model was used for determination of the solute transport. The inverse modelling tool was used to calibrate K s , α and n, and the solute transport parameter, dispersivity (D). The final soil parameters used in Hydrus-1D are presented in Table 1.
Table 1 Soil parameters used in Hydrus-1D
| Avg. depth | θ r | θ s | α | n | K s | l | D |
|---|---|---|---|---|---|---|---|
| 0.15 m | 0.09 | 0.57 | 0.03 | 1.53 | 17 | 0.5 | 15 |
| 0.45 m | 0.09 | 0.57 | 0.02 | 1.4 | 65 | 0.5 | 14 |
| 0.75 m | 0.08 | 0.56 | 0.015 | 1.3 | 10 | 0.5 | 14 |
| 1.05 m | 0.08 | 0.55 | 0.01 | 1.1 | 65 | 0.5 | 25 |
| 1.35 m | 0.08 | 0.55 | 0.01 | 1.1 | 25 | 0.5 | 25 |
The crop evapotranspiration (ET c ) was calculated according to the single crop coefficient approach proposed by Allen et al. (1998), in which ET c , is calculated by multiplying the reference crop evapotranspiration (ET o ), by a crop coefficient, K c . ET o was calculated using the Penman-Monteith equation with data from a nearby meteorological station. The K c was set to 0.4 for the initial development and 0.85 in the developed phase, with the cycle length adapted to site conditions. As required by Hydrus-1D, ET c was fractioned in the components of soil evaporation and potential crop transpiration, which were estimated as a function of the Leaf Area Index (LAI) and the corresponding Soil Cover Factor, according to the approach proposed by Ritchie (1972). The LAI of the developed pasture was 3.5. In Hydrus-1D, T was integrated over the root domain using the Feddes model for root water uptake with the parameters for pasture proposed by Wesseling et al. (1991) and the solute stress was considered using the multiplicative threshold model.
The calibration of the model with the field data resulted in a RMSE of calibration of 0.064 for a θ range between 0.2 and 0.55 and a RMSE of 6.5 for a EC sw range between 5 and 70 dSm-1.
Simulation of the climate scenarios
Hydrus-1D was also used to simulate θ and EC sw for three scenarios comprised by 30-years periods: reference, RCP 4.5, and RCP 8.5. The reference scenario corresponds to the model data for the historical period of 1971-2000. RCP 4.5 and RCP 8.5 represent different levels of greenhouse gas concentration in the atmosphere during the period 2041-70, corresponding to an intermediate and a severe scenario, respectively. The climate data was obtained from the CORDEX experiment, with the Regional Climate Model, RACMO22E (Meijgaard et al., 2008), forced by the Global Climate Model, MOHC-HadGEM2-ES. This combination was found to have better results in climate description for this region of Portugal (Soares et al., 2017).
ET c was calculated as described for the calibration process, but ET 0 was now calculated using the climate data from the projection model, using the Hargreaves-Samani equation (Hargreaves & Samani, 1985):
where C is the radiation adjustment coefficient [°C-0.5], Ra is extraterrestrial solar radiation [MJ], λ is the latent heat of vaporization equal to 2.45 MJkg-1, tavg is the average daily temperature, and t min and t max are respectively, the minimum and maximum daily temperatures [°C]. For ‘interior’ locations, where land mass dominates and air masses are not strongly influenced by a large water body (Allen et al., 1998) proposed C=0.16. t min , t max , and the precipitation, were obtained from the climate projections. The depth and EC of ground water was considered content during the simulations (1.2 m), which is an approximation, as its depth varies during the year and is also likely to rise as a consequence of the projected increase in sea water level.
RESULTS
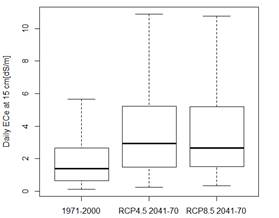
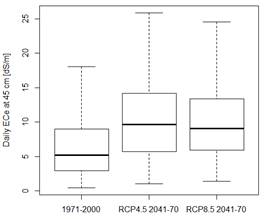
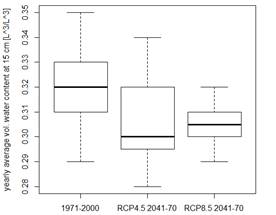
Because EC e allows the analysis of the soil salinity independently of its actual θ, it was calculated using EC sw and θ simulated with Hydrus-1D in eq. (1), where SP is θ s . Figures 1 and 2 show that the median of EC e increases in both RCP scenarios, when compared to the reference scenario. Furthermore, there is a very large increment in the maximum EC e in RCP scenarios, reaching values only tolerable to few plants. EC e shows a similar increase for the deeper soil layers. Figure 3 show the yearly average θ at 15 cm. In the topsoil a decrease in average θ in the RCP scenarios compared to the reference scenario is evident. The decrease in θ does not occur at the layers below, which is likely due to the effect of capillary rise of the groundwater to these layers.
CONCLUSIONS
The simulations show that soil salinity increases (median and maximum of daily EC e ) for all soil layers, in the future climate scenarios comparatively to the reference scenario. Also, the average yearly θ of the topsoil decreases in the future scenarios, compared to the reference. Both changes in salinity and water content may limit the productivity of the pasture and even compromise the ability of plants to grow. This evidences the need for adaptation strategies to avoid degradation of these soils due to salinity.