INTRODUCTION
Brazil is currently one of the main players in agribusiness, with competitive agriculture and cattle raising, a vibrant agroindustry and sound institutions. Exports of this segment of the Brazilian economy in 2018 surpassed US$ 85 billion, with the meat complex responsible for US$ 14.7 billion (Agrostat, 2019).
However, Brazil has undergone internal and external pressure, which affects the expectations of agents and generates strong noise among producers, particularly exporters. In recent years, the beef sector has been the most affected by these exogenous shocks (generally sanitary issues on farms and in exporter cold storages, and others of political nature). In this context, the aim of this article was to determine whether the beef exports series, in US$ and in sales volume (Kg), to the main destinations showed persistence to shocks. More specifically, we sought to test for the presence of long term memory in the series, verify the existence of structural breaks in the data, and to determine whether there was heterogeneity in the behavior of the series.
The hypothesis is that the series presents persistence to shocks, i.e., that the Brazilian beef sector already has consolidated markets, it exports quality beef at a competitive price and is thus not subject to breaks in imports, in a general and persistent manner, due to problems considered exogenous. In other words, there may be debates regarding prices, but the volume exported follows the expected trends.
The contribution of this article is based on two aspects: i) the methodology used (fractional integration analysis: long memory test or persistence to shocks) which has become an alternative method to understand time series and has yet to be used in beef export series; and ii) the contribution to understanding the dynamics of the Brazilian beef export market that has been facing innumerous challenges to its position as world leader.
In addition to this introduction, the second part of the article reviews the literature, followed by the methodology and data, the results and discussion, and the concluding remarks in the fifth and last section.
REVIEW OF THE LITERATURE
In this section, we review the literature on the Brazilian beef export sector, followed by the studies that discuss the methodology used.
The global beef market
Given its strategic importance for Brazil, a large number of studies have been conducted in recent years in order to understand the determinants of the economic growth of this activity and the exports of the sector: determinants, performance, barriers and environmental implications (Miranda and Barros, 2003; Steiger, 2006; Machado et al., 2006; Silva Neto and Bacchi, 2014; Schierhorn et al., 2016; Lima, 2018).
The studies show that the supply variables (herds and productivity) have determinant behavior for the growth of Brazilian beef cattle (Silva Neto and Bacchi, 2014), a result which is, in a certain way, corroborated by Machado et al. (2006) who highlighted the variables related to infrastructure as responsible for the increase in exports. Steiger (2006) showed that in Mercosur, Brazil and Argentina are traditional export countries and that the former faces certain environmental challenges and has the advantage of subsidized credit for the growth of the meat agro-industry. Miranda and Barros (2003) addressed the impact of non-tariff barriers on beef, for a given period, and concluded that non-sanitary barriers have not produced a significant impact on the performance of the sector. The study by Schierhorn et al. (2016) showed that the collapse of the former USSR increased Russian domestic consumption of beef and that this benefitted the production and sale of Brazilian beef to that country and helped make Brazil the largest global exporter. Lima (2018) constructed a theoretical model capable of identifying the determinants of Brazilian beef exports, and the result converged with that found by Silva Neto and Bacchi (2014), but with the capturing of the impact of a variable that negatively measured the effects of external demand.
Fractional Integration
In recent years, fractional integration has become an alternative and feasible modeling method for various time series. The idea behind this specification is that dependence between observations, which are increasingly distant over time, may be better captured in terms of the hyperbolic decay rate instead of the exponential rate associated with the autoregressive structure (Gil-Alana, 2008). In other words, the presence of long memory is related to the persistence of the autocorrelation of each series (Apergis and Tsoumas, 2011, 2012) and its memory in relation to shocks. Shocks are events that occur at a specific point in the series and are not limited to said point. It is known to have a temporary or short-term effect if, after several periods, the series returns to its previous level of performance. On the other hand, shocks may have persistent or long-term effects if the short-term impact leads to and defines a new trend in the behavior of the series (Barros et al., 2016).
No studies were found in the agricultural and livestock time series literature addressing the scope proposed here. However, in other fields of study in economics the method is more widespread, and is summarized below. In energy economics, for example: it is well known that series may present trends, seasonality, absence of linearity, fractal structures, among other characteristics; studies by Chen and Lee (2007), Narayan and Smyth (2007, 2008), Hsu et al. (2008), Maslyuk and Smyth (2009) and Mishra et al. (2009), for example, use a variety of unit root test procedures based on the I(0)/I(1) dichotomy, i.e., stationary/stationary in the first differences, to study energy consumption and production. Other studies, including Narayan et al. (2010), Apergis and Payne (2010), Aslan (2011), Aslan and Kum (2011), Hasanov and Telatar (2011), Ozturk and Aslan (2011) and Kula et al. (2012) use conventional unit root and stationarity tests to analyse the integration properties of various types of energy consumption. However, the conventional unit root tests have low power when the process is long memory (Fava and Alves, 1998), since they are not able to distinguish the I(1) processes from the fractional integration processes, I(d). It therefore becomes necessary to apply own methods to evaluate fractionally integrated series when long memory is suspected.
Several methodologies may be used to study the long term memory of a series (Souza et al., 2006), and include the classic R/S, of Hurst (1951) and Mandelbrot (1972), the modified R/S analysis, of Lo (1991) (see Tabak and Cajueiro, 2007), the fractional integration estimation parameter proposed by Geweke and Porter-Hudak (1983), the semiparametric by log-periodogram estimator, of Robinson (1995a), the Gaussian semiparametric estimator, of Robinson (1995b), and the V/S analysis, developed by Giraitis et al. (2003) and Cajueiro and Tabak (2005). The present study is similar to studies conducted by Lean and Smyth (2009), Gil-Alana et al. (2010), Apergis and Tsoumas (2011, 2012), Barros et al. (2011, 2012, 2016), which used the fractional integration methodology to identify the degree of persistence of the series, particularly the study by Barros et al. (2012), who used the method proposed by Robinson (1995a) to estimate the fractional parameter.
METHODOLOGY AND DATA
Method
In order to attain the aims proposed by this study, each of the beef export series is denoted by y t and its behavior is described by the following model:
(1)
where 𝛽 é is the vector of unknown coefficients (𝑘 x 1), 𝑍 𝑡 is a set of deterministic terms which may include an intercept ( 𝑍 𝑡 =1), and intercept with and linear time trend ( 𝑍 𝑡 = (1,𝑡) 𝑇 ), or any other type of deterministic process, and the determinists and 𝑥 𝑡 are the regression errors.
According to Barros et al. (2012), the time series 𝑥 𝑡 (𝑡=1, 2, …) is fractionally integrated to the d order, and follows a model 𝐼(𝑑) represented by:
(2)
where 1−𝐿 𝑑 is the fractinal difference operator, 𝐿 is the lag operator (i.e., 𝐿𝑥 𝑡 = 𝑥 𝑡−1 ), 𝑑 is the integration order of the process, which may be any real number, and 𝑢 𝑡 is a stationary process 𝐼(0), with mean zero and 𝑓 𝑢 (𝜆) spectrum.
The polynomial (1−𝐿) 𝑑 on the left side of Equation (2) may be expressed in terms of binomial expansion for any real number 𝑑, by:
In other terms,
Barros et al. (2011, 2016) pointed out that the parameter 𝑑, represented by equation (2), plays a crucial role in the data analysis because it is an indicator of the degree of independence of the series. The higher the value of 𝑑, the higher the level of association between the observations that are increasingly distant in time.
In the case of 𝑑=0 in (2), the stochastic process 𝑥 𝑡 has stationary covariance. If the fractional parameter assumes the value of 𝑑=1, 𝑥 𝑡 is a non-stationary process with unit root, i.e., the model contains a stochastic trend. Thus, the fractional integration arises when 𝑑 assumes positive non-whole values, 0<𝑑<1. If the value of 𝑑 is restricted to the interval 0<𝑑<0.5, 𝑥 𝑡 is mean-reverting and continues to be a stationary covariance process, but with a slower autocovariance function decay than the stationary case, 𝐼(0). If 0.5≤𝑑<1, 𝑥 𝑡 is non-stationary, the mean reversion and its autocovariance function are more persistent (Apergis and Tsoumas, 2011, 2012). However, if 𝑑≥1, 𝑥 𝑡 is non-stationary and non-mean reverting (Gil-Alana, 2008).
Processes with 𝑑>0 in equation (2) exhibit the property of "long memory", which is given this name due to the strong degree of association between observations that are very distant in time (Barros et al., 2016). The responses to the impulse are also affected by the magnitude of 𝑑, and, according to Barros et al. (2011), the higher the value of 𝑑, the higher the responses. In the case of 𝑑<1, the series is mean reverting with shock from temporary effects disappearing in the long term. However, if 𝑑≥1, the shock will have permanent effects, unless strong political measures are taken.
In the context of fractional processes, Gil-Alana (2008) states that the occasional disregard of structural breaks may lead to the spurious discovery of long memory. Therefore, in this study we examine the possibility of fractional integration in the presence of a single structural break at an unknown point in the sample. Thus, each one of the series, 𝑦 𝑡 , which presents a break is represented by the following model:
(3)
(4)
where the β’s are the coefficients corresponding to the deterministic terms, 𝑑 1 and 𝑑 2 are real numbers, 𝑢 𝑡 is a stationary 𝐼(0) process, with mean zero and spectrum 𝑓 𝑢 (𝜆), and 𝑇 𝑏 is the unknown break point.
The methodology used in this study to estimate the fractional differentiation parameter is the method proposed by Robinson (1995a), the Gaussian Semiparametric Estimator (GSE) based on the Whittle Function in the frequency domain. The test proposed by Andrews and Ploberger (1994) was also applied, with p-value assuming the approximations of Hansen (1997), recommended to test a single structural rupture at an unknown point within the sample and to identify the date of the break, 𝑇 𝑏 . New estimates are then made of the parameter d in the presence of the break .
Data
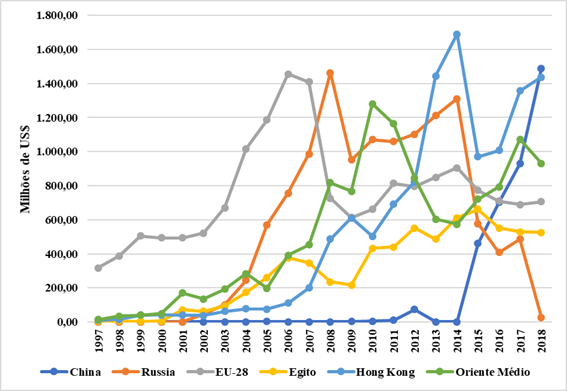
The annual series of Brazilian beef exports to the main destinations were collected from Agrostat (2019) (in 2018 the value was slightly higher than US$ 5 billion) between 1997 and 2018 (Figures 1 and 2).

Figure 1 Brazilian beef exports (in pounds), main destinations (1997-2018). Source: Agrostat (2019).
Figures 1 and 2 show that the various units of measurement had the same behavior, which may indicate potential acceptance of the hypothesis put forth by this study that expected the possibility of minimum difference between the volume and the negotiated value series, where the noise on the market only indicated the seeking of price bargaining opportunities. This hypothesis, however, will be proven with the series memory tests.
The foot-and-mouth disease outbreak in Europe in 2005 significantly affected the exports of this economic bloc, probably due to the fear consumers had of ingesting beef. Another aspect to be highlighted is the exceptional growth in exports to China as of 2014.
Furthermore, an important fact is the recognition of Brazil, as of 2003, as the main global beef exporting country, surpassing Australia and the United States (Silva Neto, 2011; Lima, 2018).
RESULTS
In Tables 1 and 2 we present the results of the fractional parameter estimations. The second column lists the estimates of the Robinson (1995a) d parameter for the beef export series, in currency (US$) and sales volume (Kg), for the main destinations. Disregarding the possibility of a structural break, the estimates of the model’s d parameter, given by equations (1) and (2) show that for the series in US$, China, Egypt and the Middle East showed estimates within the interval (0,1). Regarding the series in Kg, only China had an estimate within this interval, 𝑑= 0.6993. This suggests that they are fractionally integrated.
Table 1 Estimation of the fractional parameter of the beef export series - main destinations (US$)
| Entire sample | Structural Break | Before the break | After the break | |||||||
| d * | std error | d 1 | std error | t 1 | d 2 | std error | t 2 | |||
| China | 0.5960 | 0.1508 | 2014 | 0.5807 | 0.1581 | 18 | 6.6813 | 0.2887 | 4 | |
| Russia | 1.3889 | 0.1581 | 2003 | 1.5000 | 0.3536 | 3 | 1.3158 | 0.1768 | 15 | |
| UE 28 | 1.0424 | 0.1508 | 2002 | 2.0662 | 0.2500 | 6 | 1.8066 | 0.1667 | 16 | |
| Egypt | 0.9608 | 0.1508 | 2000 | 7.8079 | 0.2887 | 4 | 0.9139 | 0.1581 | 18 | |
| Hong Kong | 1.2005 | 0.1508 | 2006 | 1.5943 | 0.2041 | 10 | 1.4388 | 0.1890 | 12 | |
| Middle East | 0.9727 | 0.1508 | 2002 | -2.2894 | 0.2500 | 6 | 0.6250 | 0.1667 | 16 | |
Source: Prepared by the authors.
d = fractional parameter of the entire sample; 𝑑 1 = fractional parameters before the break; 𝑡 1 = observations before the break; 𝑑 2 = fractional parameters after the break; 𝑡 2 = observations after the break
Table 2 Estimation of the fractional parameter of the beef export series - main destinations (Kg)
| Entire sample | Structural Break | Before the break | After the break | |||||||
| d | std error | d 1 | std error | t 1 | d 2 | std error | t 2 | |||
| China | 0.6993 | 0.1508 | 2014 | 0.7641 | 0.1581 | 18 | 2.5659 | 0.2887 | 4 | |
| Russia | 1.3751 | 0.1581 | 2003 | 1.4500 | 0.3536 | 3 | 1.3773 | 0.1768 | 15 | |
| EU 28 | 1.7550 | 0.1508 | 2007 | 1.3265 | 0.2500 | 6 | 1.9702 | 0.1667 | 16 | |
| Egypt | 1.0555 | 0.1508 | 2000 | 5.9138 | 0.2887 | 4 | 1.0903 | 0.1581 | 18 | |
| Hong Kong | 1.2413 | 0.1508 | 2006 | 1.7974 | 0.2041 | 10 | 1.6253 | 0.1890 | 12 | |
| Middle East | 1.0984 | 0.1508 | 2000 | -1.7683 | 0.2500 | 6 | 1.0277 | 0.1667 | 16 | |
Source: Prepared by the authors.
d = fractional parameter of the entire sample; 𝑑 1 = fractional parameters before the break; 𝑡 1 = observations before the break; 𝑑 2 = fractional parameters after the break; 𝑡 2 = observations after the break
The four series mentioned above have a high degree of persistence, 0.5≤𝑑<1, with less expressive fluctuations when compared with series with lower persistence, 0<𝑑<0.5. The other series in US$ (Russia, EU 28 and Hong Kong) and in Kg (Russia, EU 28, Egypt, Hong Kong and the Middle East) have values greater than 1, indicating that a shock in the series itself will have permanent effects, unless strong policy measures are taken. In other words, the hypothesis of the study is accepted: the Brazilian beef export market is internationally consolidated, with a consistent volume, an adequate range of countries, and market fluctuations that do not seem to affect exports in a persistent manner.
In light of the possibility of a structural break generating inconsistent fractional parameter estimates (Lima et al., 2015), the Andrews and Ploberger (1994) test was conducted, using the p-value approximations of Hansen (1997). This test was used to identify potential structural changes in the data, thus every ( 𝑦 𝑡 ) containing a break assumes the model represented by equations (3) and (4). Thus, the break points ( 𝑇 𝑏 ) are in the fourth column, the values before the break ( 𝑑 1 ) in the fifth column, and the eight column lists the values after the break ( 𝑑 2 ).
The results of the procedure indicate that the 12 series have a structural change. Of the six main destinations, four presented the same break date for beef exports in currency and in volume sold - China (2014): the suspension of the trade embargo imposed as of 2012 after the foot-and-mouth disease in the state of Paraná; Russia (2003): the establishing of quotas to protect local producers; Egypt (2000): with the “mad cow” crisis in Europe, the country resorted to the Brazilian market; and Hong Kong (2006): foot-and- mouth disease outbreak in 2005 in the states of Paraná and Mato Grosso do Sul caused a strict embargo on Brazilian beef, with the exception of Hong Kong that maintained the trade.
The series that contained breaks were divided into two samples, as per equations (3) and (4), the first sample to estimate parameter 𝑑 1 with size 𝑡 1 =1, 2, …, 𝑇 𝑏 , and the second to estimate 𝑑 2 with size 𝑡 2 = 𝑇 𝑏 +1, …, 𝑇. Since the period under study was 1997-2018, this procedure led some samples to contain few observations, thus corroborating the inconsistent values of 0>𝑑 1 , 𝑑 2 >2. In these cases, they were not considered in the study. The total observations before and after the break, 𝑡 1 and 𝑡 2 , are in the seventh and tenth columns (Tables 1 e 2).
The d parameters estimated for the period prior to the break confirmed the persistence of the US$ and Kg series for China, 0.5≤𝑑<1, presenting non-stationary behavior, but with mean reversion. The 𝑑 1 of the remaining series were all above the unit. However, the post-break period highlights the persistence of the US$ series for Egypt and the Middle East, while the others had 𝑑≥1, which indicates non-stationary and non-mean reverting behavior.
The analysis of the degree of persistence to shocks allows identifying the presence of long memory in the beef export series to the main destinations. Long memory may be regarded as a problem only in cases where the value of beef exports, in monetary values or in amount sold to the main destinations, is low; otherwise it should be considered an advantage. In other words, the value and amount exported in the current period is the result of a series of past events but which continue to influence future periods. Therefore, if, for a previous period, the volume of beef exports, in US$ or in Kg, were high, the volume will also tend to be high in the subsequent period. This demonstrates that Brazilian beef has a strong presence on the international market and that it is not susceptible to exogenous shocks: political interference, specific sanitary problems, trade embargoes, and others.
CONCLUDING REMARKS
The present study analysed persistence in beef export series, in currency (US$) and volume sold (Kg), to the main destinations - China, Russia, EU 28, Egypt, Hong Kong and the Middle East - aiming to test for the presence of long memory in the series, verify the existence of structural breaks in the data, and to identify whether there was heterogeneity in the behavior of the US$ and Kg.
With regard to heterogeneity in the behavior of the series, the results indicate that the degree of persistence changed from one series to the other, depending on the type, in US$ or Kg, and the destination of the exports. However, in certain cases, the fractional differentiation parameter values for the same destination, in US$ and Kg, were similar.
In most of the series, the integration order was greater than the unit, mainly for the Kg series, indicating non-stationary and non-mean reverting behavior. While the other export series had long memory, in these cases they were not stationary, but were mean reverting over time. The persistence of these series is related to the time they took to return to their previous level of behavior in the presence of exogenous shocks. According to Barros et al. (2012), understanding the degree of persistence of a series is relevant to the policy to be implemented over time.
Considering the potential presence of structural breaks in the data, few significant changes were found in the integration order, thus confirming the presence of long memory in the series for China (US$ and Kg), Egypt (Kg), and the Middle East (Kg). The remaining series showed d estimates greater than the unit, indicating non-stationary and non-mean reverting behavior.
According to the results it is possible to conclude that Brazil has a consolidated beef export market, which is strong and resistant to exogenous shocks in either the domestic market or from abroad. Its leadership is consolidated and tends to provide quality beef to all economic blocs. In addition, the country has the structural conditions to become the world’s main animal protein supplier.
Further research may be conducted to apply the methodology used here to the pork and poultry markets, which are equally important Brazilian exports. Other commodities, such as soybean, ethanol, coffee and others may also be objects of study based on this article.